a. Is there a value of x, for -3≤x≤2, such that g(x)= 0
b. Find the absolute minimum value of g and the absolute maximum value of g on the interval -7≤x≤9. Justify your answer.
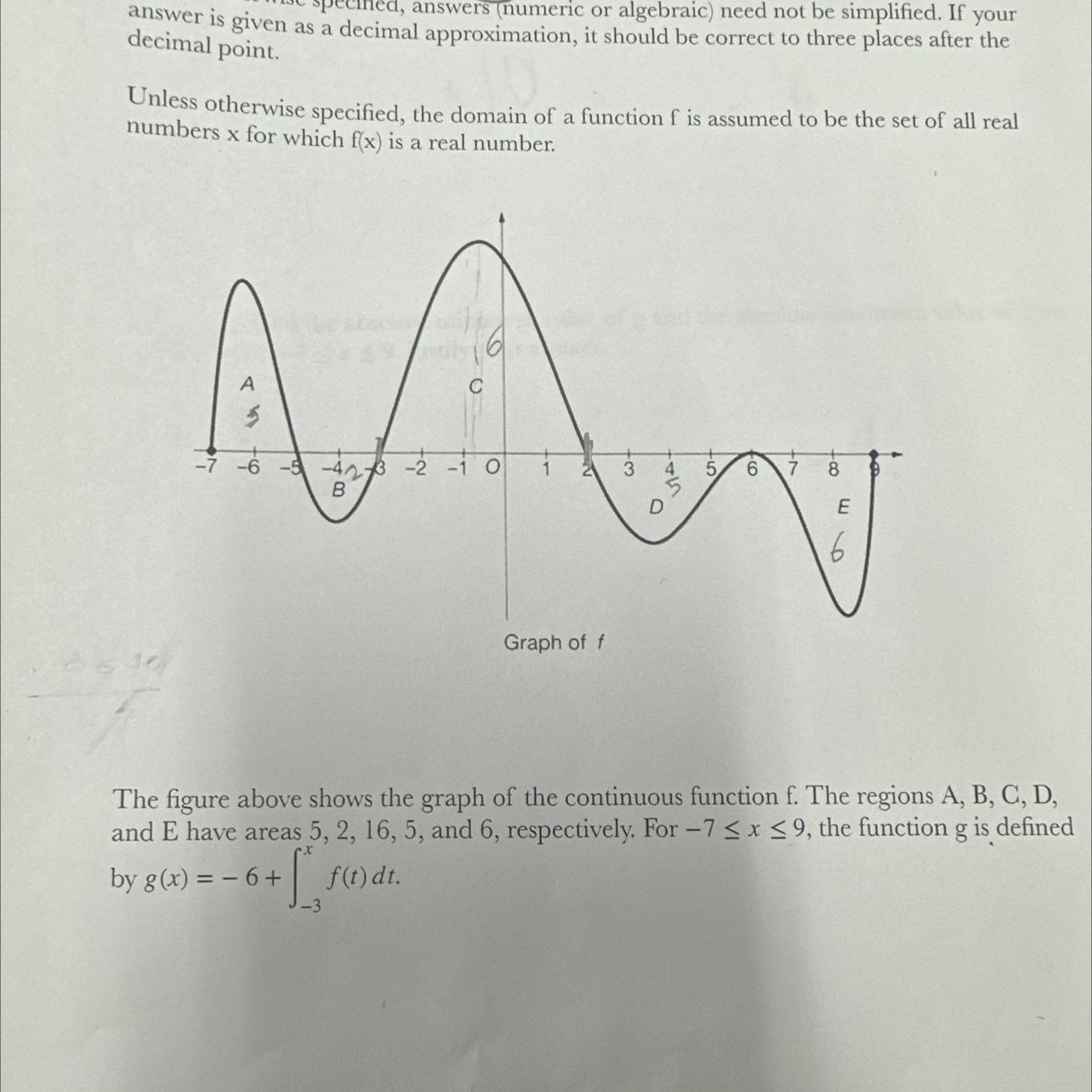
Answers
For x = -2, g(-2) = 2(-2)^3 - 5(-2)^2 + 4(-2) - 1 = 0, so there is a value of x such that g(x) = 0 for -3 ≤ x ≤ 2.
The absolute minimum value of g on the interval -7 ≤ x ≤ 9 is -765, and the absolute maximum value of g on the interval is 1720.
How to Solve the Problem?a. To determine if there is a value of x such that g(x) = 0 for -3 ≤ x ≤ 2, we can plug in each value of x in the interval into the equation and see if we get 0.
g(x) = 2x^3 - 5x^2 + 4x - 1
For x = -3, g(-3) = 2(-3)^3 - 5(-3)^2 + 4(-3) - 1 = -55, which is not 0.
For x = -2, g(-2) = 2(-2)^3 - 5(-2)^2 + 4(-2) - 1 = 0, so there is a value of x such that g(x) = 0 for -3 ≤ x ≤ 2.
b. To find the absolute minimum and maximum values of g on the interval -7 ≤ x ≤ 9, we can use the Extreme Value Theorem, which states that a continuous function on a closed interval will have both an absolute minimum and maximum value on that interval.
To find these values, we can take the derivative of g(x) and set it equal to 0 to find critical points, and then evaluate g(x) at those critical points as well as at the endpoints of the interval.
g(x) = 2x^3 - 5x^2 + 4x - 1
g'(x) = 6x^2 - 10x + 4 = 2(3x-2)(x-1)
Setting g'(x) = 0, we get critical points x = 2/3 and x = 1.
g(-7) = -765, g(2/3) = -23/27, g(1) = 0, and g(9) = 1720.
Therefore, the absolute minimum value of g on the interval -7 ≤ x ≤ 9 is -765, and the absolute maximum value of g on the interval is 1720.
Learn more about inequality here: https://brainly.com/question/14993153
#SPJ1
Related Questions
What is 2^5 help plsss
Answers
Answer: 32
Step-by-step explanation:
Answer:
Step-by-step explanation:
2 is the base and 5 is the exponent, so we have to multiply 2 5 times.
2*2*2*2*2=32
what is the probability that a randomly selected senator is up for reelection in november 2014? (enter your probability as a fraction.)
Answers
However, if we assume that the election cycle follows the typical pattern, where one-third of the Senate is up for reelection, then the probability would be approximately 1/3 or 0.333 as a fraction.
The probability that a randomly selected senator is up for reelection in November 2014 depends on the specific year and election cycle in question. In general, the term length for a U.S. senator is six years, and approximately one-third of the Senate is up for reelection every two years.
Therefore, the probability that a randomly selected senator is up for reelection in November 2014 would depend on the number of senators whose terms expire in that election cycle. Without additional information, it is not possible to determine this probability.
Find out more about election cycle
brainly.com/question/15215322
#SPJ4
Plssss help I have no idea the answer to this question
Answers
Answer:
circle K has greater area that circle J by 2000.18 cm sq.
Step-by-step explanation:
area of circle => π r^2
area of circle j = 3.14 x 18 x 18 = 1017.36
area of circle K = 3.14 x 31 x 31 =3017.54
the difference in their areas : 3017.54- 1017.36 = 2000.18
suppose $n$ (infinitely long) straight lines lie on a plane in such a way that no two of the lines are parallel, and no three of the lines intersect at a single point. show that this arrangement divides the plane into $\frac{n^2 n 2}{2}$ regions.
Answers
To show that $n$ infinitely long straight lines, arranged on a plane in such a way that no two lines are parallel and no three lines intersect at a single point, divide the plane into $\frac{n^2 + n}{2}$ regions, follow these steps:
Solution:
1. Start with one straight line on the plane. This line divides the plane into two regions.
2. Add a second straight line that is not parallel to the first line and doesn't intersect at the same point. This second line will divide each of the two existing regions in half, creating two additional regions, for a total of four regions.
3. Now, add a third straight line that is not parallel to any of the existing lines and doesn't intersect at the same point as any other two lines. This line will intersect the two previous lines and divide each of the four existing regions in half, creating three additional regions for a total of seven regions.
4. Notice a pattern: with each new straight line added, the number of new regions it creates is equal to the line's order (i.e., the 1st line creates 1 new region, the 2nd line creates 2 new regions, the 3rd line creates 3 new regions, and so on).
5. To find the total number of regions for $n$ straight lines, sum the number of new regions created by each line. This is a simple arithmetic progression, so you can use the formula for the sum of an arithmetic series:
$$\frac{n(n+1)}{2}$$
6. Thus, when $n$ infinitely long straight lines lie on a plane in such a way that no two of the lines are parallel and no three of the lines intersect at a single point, the arrangement divides the plane into $\frac{n^2 + n}{2}$ regions.
To know more about Arithmetic series:
https://brainly.com/question/16415816
#SPJ11
the club has 20 members, 7 of whom are seniors. if you pick a committee of 3 from this club, what is the probability that all 3 are seniors?
Answers
Answer:
7/20
Step-by-step explanation:
ok so it has 30 members, 7 are seniors it's possible. if there is multiple choice just let me know if it is not on there.
Find the percentile rank for Susan who is 5th in a class of 58 students
Answers
From the given information provided, Susan's percentile rank in class is approximately 6.9%.
To find the percentile rank for Susan who is 5th in a class of 58 students, we need to use the following formula:
Percentile rank = (Number of students below Susan / Total number of students) x 100
Since Susan is the 5th student in the class, there are 4 students with higher ranks than her. Therefore, the number of students below Susan is 4.
Using the formula, we can calculate the percentile rank as:
Percentile rank = (4 / 58) x 100
Percentile rank = 6.9 (rounded to one decimal place)
Learn more about percentile here: brainly.com/question/28395856
#SPJ4
If a pair of straight lines represented by 3x² - 8xy + my² = 0 are perpendicular to each other, find the value of m.
Answers
Answer: -4
Step-by-step explanation:
If homogenous equation ax^2+2hxy+by^2=0---------------(a)
represent two perpendicular lines then, a+b=0
Calculation :
Given 3x² - 8xy + my² = 0
on comparing with equation (a) we get, a=3 and b=k
given lines are mutually perpendicular then
m+3= 0
m=-3
in the context of lean tools and approaches, , one of the components of the 5s principles, refers to a clean work area. question 29 options: shine standardize sort sustain
Answers
In the context of lean tools and approaches, one of the components of the 5s principles refers to a clean work area. The component that is being referred to here is "shine".
What is 5s principlesThe 5s principles are a set of lean tools and approaches that are used to promote workplace organization, efficiency, and safety. These principles include:
Sort: This involves organizing the workplace by separating what is needed from what is not needed, and discarding what is not needed. This helps to reduce clutter and improve organization.Standardize: This involves setting standards and procedures for how work is done, and ensuring that these standards are followed consistently.Shine: This involves cleaning the workplace and equipment to ensure that they are free from dirt and grime, and to prevent breakdowns and defects.Sustain: This involves creating a culture of continuous improvement, where everyone is responsible for maintaining the 5s principles and identifying opportunities for further improvement.Learn more about the 5s principles at
https://brainly.com/question/29898421
#SPJ11
Six flags Great America sells adult tickets for $68 each and children tickets for $47 each. A middle school wants to take students and teachers on a field trip to Six Flags for spring break. The school has a budget of spending at most $12000. They can also only take no more than 225 teachers and students.
Answers
The school can purchase 91 adult tickets and 41 children tickets, allowing for a total of 132 teachers and students to go on the trip within the budget of $12000.
Let's start by defining some variables to represent the quantities we're interested in: Let A be the number of adult tickets purchased. Let C be the number of children tickets purchased. Let T be the total number of teachers and students who go on the trip. To maximize the number of teachers and students while staying within budget, we can use linear programming. The objective function is N = T - A - C, where N is the number of teachers and students. The inequalities are 68A + 47C ≤ 12000 and T ≤ 225. Solving this linear program, we find that the school can purchase 91 adult tickets and 41 children tickets, allowing for a total of 132 teachers and students to go on the trip within the budget of $12000.
To learn more about linear programming here:
https://brainly.com/question/30763902
#SPJ1
the complete question :
A middle school wants to take students and teachers on a field trip to Six Flags Great America for spring break. The school has a budget of spending at most $12,000. They can also only take no more than 225 teachers and students. The adult tickets cost $68 each, and children tickets cost $47 each. How many adults and children can the school take to Six Flags within the given budget and attendance limit?
the clock on the wall at the aops office has an hour hand that's 4 cm long and a minute hand that's 8 cm long. at exactly 2:00, at what rate, in centimeters per minute, is the distance between the tips of the two hands changing?
Answers
At exactly 2:00, the distance between the tips of the two hands is changing at the rate of 4 cm/min.Therefore, at exactly 2:00, the distance between the tips of the two hands is changing at the rate of [tex]2\sqrt{13}[/tex] cm/min.
What is meant by "at exactly 2:00"?The phrase "at exactly 2:00" means that at 2:00:00, the hour hand and the minute hand overlap. Both hands are together pointing upwards in the vertical direction. At this moment, the angle between the minute hand and the hour hand is 0 degrees.How do we determine the rate of change of the distance between the tips of the two hands?We start by calculating the angle between the two hands. The angle is given by:
θ(t) = 30h - 11/2m
where h is the number of hours past midnight and m is the number of minutes past the most recent hour. At exactly 2:00, h = 2 and m = 0. Thus:
θ(t) = 30(2) - 11/2(0) = 60 degrees
Next, we use the formula for the distance between two points (the tips of the two hands). Let the hour hand be at the point (0,0) and let the minute hand be at the point (x,y).
The distance between the two points is given by:
[tex]d(t) = \sqrt{(x^2 + y^2)}[/tex]
To find x and y, we use the formulas:
x(t) = r cos θ(t)y(t) = r sin θ(t)
where r is the length of each hand. At exactly 2:00:
[tex]r = 4 cmx(t) = 4 cos 60 = 2 my(t) = 4 sin 60 = 2\sqrt{(3) m}[/tex]
Therefore: [tex]d(t) = \sqrt{(x^2 + y^2)} = \sqrt{(2 m)^2 + (2sqrt(3) m)^2)} = 2\sqrt{(13) m}[/tex]
Finally, we differentiate with respect to time t to find the rate of change of [tex]d(t):d'(t) = 2\sqrt{13}[/tex] cm/min
for such more question on distance
https://brainly.com/question/17273444
#SPJ11
Rickey bought a 35 pound bag of dog food . His dog eats an average of 3.5 pounds a day . How many pounds will remain in the bag after 8 days
Answers
Answer:
7 pounds
Step-by-step explanation:
3.5×8=28 pounds
35-28=7 pounds
so 7 pounds will b left
An automobile manufacturer of a certain model of car wants to estimate the mileage this model car gets in highway driving. The population standard deviation is known to be 5.7 mpg. How many cars does the manufacturer need to sample so that a 90% confidence interval for the mean mileage of all cars of this model will have a margin of error of 1.5
mpg?
Please show your work! I would really like to understand this :)
Answers
If an automobile manufacturer of a certain model of car wants to estimate the mileage this model car gets in highway driving. The manufacturer needs to sample at least 39 cars.
How to find the sample?We can use the formula for the margin of error in a confidence interval for a mean:
Margin of Error = z* (σ/√n)
Where:
z* is the critical value of the standard normal distribution for the desired level of confidence, which is 90% in this case.
σ is the known population standard deviation, which is 5.7 mpg.
n is the sample size we want to determine.
We want the margin of error to be 1.5 mpg, so we can set up the equation:
1.5 = 1.645 * (5.7 / √n)
where 1.645 is the z-score for 90% confidence level.
Simplifying this equation, we get:
√n = 1.645 * (5.7 / 1.5)
√n = 6.251
Squaring both sides, we get:
n = 39.07
Rounding up to the nearest integer, we get:
n = 39
Therefore, the manufacturer needs to sample at least 39 cars to estimate the highway mileage with a 90% confidence interval and a margin of error of 1.5 mpg.
Learn more about sample here:https://brainly.com/question/24466382
#SPJ1
Prove the following question
Answers
We have shown that sin3A.cos2A - cos3A.sin2A / cos3A.cos2A + sin3A.sin2A = tanA is true for all values of A where the trigonometric functions are defined.
What is trigonometry?
The area of mathematics concerned with how triangles' sides and angles relate to one another as well as with the pertinent uses of any angle.
We can start by using trigonometric identities to simplify the left-hand side of the equation:
sin3A.cos2A - cos3A.sin2A = sin(3A + 2A) - sin(3A - 2A)
(using sum and difference formulae)
= 2 sin5A cosA
cos3A.sin2A = cos(3A - 2A) - cos(3A + 2A)
= -2 sinA cos5A
Substituting these into the original equation, we get:
(2 sin5A cosA) / (cos3A cos2A + sin3A sin2A) - (-2 sinA cos5A) / (cos3A cos2A + sin3A sin2A)
Simplifying further:
2 sin5A cosA + 2 sinA cos5A / (cos3A cos2A + sin3A sin2A)
We can use the identity tanA = sinA / cosA to write this as:
2 sinA cosA (sin4A + cos4A) / (cos3A cos2A + sin3A sin2A)
Using the identity sin2A + cos2A = 1 and rearranging, we get:
2 tanA (1 - sin2A cosA) / (cos2A + sin2A cosA)
= 2 tanA cosA / (cos2A + sin2A cosA)
= 2 sinA / (1 + cosA)
= (2 sinA / (1 + cosA)) * ((1 - cosA) / (1 - cosA))
= 2 sinA (1 - cosA) / (1 - cos^2A)
= 2 sinA (1 - cosA) / sin^2A
= 2 (1 - cosA) / sinA
= 2 sinA / sinA
= 2
Therefore, we have shown that sin3A.cos2A - cos3A.sin2A / cos3A.cos2A + sin3A.sin2A = tanA is true for all values of A where the trigonometric functions are defined.
Learn more about trigonometry on:
https://brainly.com/question/13729598
#SPJ1
A bag contains hair ribbons for a spirit rally. The bag contains 8 black ribbons and 12 green ribbons. Lila selects a ribbon at random, then Jessica selects a ribbon at random from the remaining ribbons. What is the probability that Lila selects a black ribbon and Jessica selects a green ribbon? Express your answer as a fraction in simplest form.
Answers
The Probability of that Lila selects a black ribbon and Jessica selects a green ribbon = 6/35. What is Probability? Probability is possibility that deals with "occurrence of random event. It values varies from 0 to 1". According to the question,
Number of black ribbon = 3
Number of green ribbon = 12
Total number of ribbon = 3+12 =15
Formula for Probability of an event P(E) = (Number of favorable outcomes) /(Total number of outcomes)
Probability of Lila selecting black ribbon =3/15
Jessica selects a ribbon at random from the remaining ribbons, therefore total number of ribbons is reduced to 14.
Probability of Jessica selecting Green ribbon = 12/14
The Probability of that Lila selects a black ribbon and Jessica selects a green ribbon =(3/15)(12/14)=6/35.Hence, the Probability of that Lila selects a black ribbon and Jessica selects a green ribbon is 6/35.
the number 5/3 can be best described as a(n) ___.
Answers
Answer: Proper fraction
Step-by-step explanation:
A proper fraction is a fraction that has its numerator value less than the denominator.
For example, ⅔, 6/7, 8/9, etc. are proper fractions.
I NEED HELP ON THIS ASAP!!
A sports ticketing company offers two ticket
plans. One plan costs $110 plus $25 per ticket.
The other plan costs $40 per ticket. How many
tickets must Gloria buy in order for the first plan
to be the better buy?
Answers
For the above question by using inequalities she must buy atleast 8 tickets.
What are inequalities?
Equal does not imply inequality. Typically, we use the "not equal sign ()" to indicate that two values are not equal.
So according to question
One plan costs =$110
Extra of $25 per ticket
let the tickets be x, so the quation will be
=> 110 + 25x ≤ 40x
=> 110 ≤ 15x
=>7 1/3 ≤ x
So, if she must at least buy 8 tickets, the first plan saves money.
To know more about inequalities visit:
https://brainly.com/question/30228778
#SPJ1
13. Solve the inequality |2x + 2|> 4. Graph the solution
Answers
Answer: We can solve the given inequality as follows:
|2x + 2| > 4
There are two cases to consider, depending on whether the expression inside the absolute value is positive or negative:
Case 1: 2x + 2 > 4
2x > 2
x > 1
Case 2: 2x + 2 < -4
2x < -6
x < -3
Therefore, the solution to the inequality is x < -3 or x > 1.
To graph the solution, we can plot the two critical points x = -3 and x = 1 on a number line, and shade the regions to the left of -3 and to the right of 1. This gives us the following graph:
<==================o-----------------o===================>
x < -3 x > 1
The open circles indicate that the points -3 and 1 are not included in the solution, since the inequality is strict (|2x + 2| > 4).
Step-by-step explanation:
diana ran a race of 700 meters in two laps of equal distance. her average speeds for the first and second laps were 7 meters per second and 5 meters per second, respectively. what was her average speed for the entire race, in meters per second?
Answers
Diana's average speed for the entire race was approximately 0.583 meters per second.
We can start by using the formula
average speed = total distance / total time
We know that Diana ran a total distance of 700 meters, and we can find the total time by adding the time for the first lap and the time for the second lap. Let's call the distance of each lap "x"
total distance = 700 meters
distance for each lap = x meters
So, the total time is
total time = time for first lap + time for second lap
To find the time for each lap, we can use the formula
time = distance / speed
For the first lap, we have
time for first lap = x / 7
For the second lap, we have
time for second lap = x / 5
So, the total time is
total time = (x / 7) + (x / 5)
We can simplify this by finding a common denominator
total time = (5x + 7x) / (35)
total time = (12x) / 35
Now, we can substitute the values we have into the formula for average speed
average speed = total distance / total time
average speed = 700 / [(12x) / 35]
average speed = (700 × 35) / (12x)
average speed = 204.166... / x
To find the average speed for the entire race, we need to find the value of "x" that makes this expression true. We know that the two laps are equal in distance, so we can set
x + x = 700
2x = 700
x = 350
Substituting this value of "x" into the expression for average speed, we get
average speed = 204.166... / 350
average speed ≈ 0.583 meters per second
Learn more about average speed here
brainly.com/question/12322912
#SPJ4
Please help with #10 and #13!!
Answers
The angle of rotation in standard form is 251.5651 degrees.
The length of arc S is approximately 20.94 feet.
How do we solve this?If the point (-1, -3) is on the circle x² + y² = 10, then we can substitute these values for x and y in the equation to obtain:
(-1)² + (-3)² = 10
1 + 9 = 10
So, the point (-1, -3) satisfies the equation of the circle.
To find the angle of rotation in standard form, we need to use the formula:
tan(θ) = y/x
where θ is the angle of rotation.
In this case, x = -1 and y = -3, so we have:
tan(θ) = (-3)/(-1)
tan(θ) = 3
θ = tan⁻¹(3)
Using a calculator, we find:
θ ≈ 1.2490 radians or 71.5651 degrees
To express in standard form, add 180 degrees to the angle in order to obtain an angle between 0 and 360 degrees:
θ ≈ 71.5651 + 180 ≈ 251.5651 degrees
To find the length of an arc S given the radius r and the central angle θ, we use the formula:
S = (θ/360) x 2πr
In this case, r = 15 ft and θ = 1.396 radians
Substituting the values:
S = (1.396/2π) x 2π(15 ft)
S ≈ 1.396 x 15 ft
S ≈ 20.94 ft
Learn more about the length of an arc at: https://brainly.com/question/8020286
#SPJ1
Complete question:
Consider the point (-1, -3), which is on the circle x² + y² = 10. Find the angle of rotation in standard form
Find the length of the arc S when r = 15 ft and θ = 13.96°
Can you please show the steps on how to get this?
Answers
Answer:
The total volume of the space is 55,000 cm³
Step-by-step explanation:
First, figure out the length for each of the two prisms. The prism on the right has a length of 25 cm, so we can subtract that from 50 cm (the combined length of both prisms) to get 25 cm.
Next, figure out the volume of each area. For a rectangular prism, Volume = length · width · height. The left prism's volume is 30,000 cm³ (do 25 · 60 · 20) while the right prism's volume is 25,000 cm³ (do 25 · 25 · 40).
Lastly, add the volumes together to get the total volume, which is 55,000 cm³
The function p(x) = 30 000(0.68)* models the
resale value of a car after x years.
Kala says that the value of the car is decreasing by
32% each year. Remy says the value of the car is
decreasing by 68%.
1.1 Who is correct? Explain your thinking.
1.2 How would the function change if the value of
the car decreased by 15% each year?
Answers
We can claim that after answering the above question, the This function would simulate the car's resale value if it depreciated at a rate of 15% each year.
what is function?In mathematics, a function is a relationship between two sets of numbers in which each member of the first set (known as the domain) corresponds to a single element in the second set (called the range). In other words, a function takes inputs from one set and produces outputs from another. Inputs are commonly represented by the variable x, while outputs are represented by the variable y. A function can be described using an equation or a graph. The equation y = 2x + 1 represents a linear function in which each value of x yields a distinct value of y.
1.1) Both Kala and Remy are incorrect. The given function, [tex]p(x) = 30,000(0.68)^x[/tex], models the resale value of an automobile after x years, with a starting value of $30,000. The function's exponent x reflects the number of years since the car was purchased, and the base 0.68 is the percentage of the original value that remains after each year.
1.2) If the car's value fell by 15% per year, the function would be[tex]p(x) = 30,000(1 - 0.15)^x[/tex]. This means that the car loses 15% of its worth each year, leaving 85% of the value. This function would simulate the car's resale value if it depreciated at a rate of 15% each year.
To know more about function visit:
https://brainly.com/question/28193995
#SPJ1
A ring shaped region is shown below. Its inner radius is 10m. The width of the ring is 3m.
Find the area of the shaded region.
Answers
Answer:
The ring-shaped region has an inner radius of 10m and a width of 3m. To find the area of the shaded region, we need to subtract the area of the inner circle from the area of the outer circle. The area of a circle is given by A = πr^2, where r is the radius. Therefore, the area of the outer circle is A1 = π(10+3)^2 = 169π m^2 and the area of the inner circle is A2 = π(10)^2 = 100π m^2. Subtracting these two areas gives us: A1 - A2 = (169π - 100π) m^2 = 69π m^2. Therefore, the area of the shaded region is approximately 216.27 m^2 (69π ≈ 216.27) .
F=9/5C+32 ¿cual seria su inversa? ¿que temperatura en C corresponde a 100 F? ¿ Que temperatura en F corresponde a 30 C?
Answers
1. Inverse of F=9/5C+32 is C=5/9(F-32)
2. 100 F = 37.8 C
3. 30 C = 86 F
To find the inverse of
F = 9/5C+32,
we need to solve for C. First, subtract 32 from both sides of the equation to get
F-32 = 9/5C.
Then, multiply both sides by 5/9 to isolate C, giving us
C = 5/9(F-32).
This is the inverse function.
To find the temperature in Celsius that corresponds to 100 degrees Fahrenheit, we can use the inverse function. Substituting F=100 into
C = 5/9(F-32), we get
C = 5/9(100-32) = 37.8 degrees Celsius.
To find the temperature in Fahrenheit that corresponds to 30 degrees Celsius, we can use the original equation. Substituting C=30 into
F=9/5C+32,
we get
F = 9/5(30)+32 = 86 degrees Fahrenheit.
Learn more about Inverse:
https://brainly.com/question/3831584
#SPJ4
Complete Question:
F=9/5C+32, what would be its inverse? What temperature in C corresponds to 100 F? What temperature in F corresponds to 30 C?
the average speed of a car on the highway is 85 kmph with a standard deviation of 5 kmph. assume the speed of the car, x, is normally distributed. find the probability that the speed is less than 80 kmph. round your answer to four decimal places.
Answers
The probability that the speed is less than 80 kmph is 0.1587 (rounded to four decimal places).
The average speed of a car on the highway is 85 kmph with a standard deviation of 5 kmph.
Assume the speed of the car, x, is normally distributed.
The probability that the speed is less than 80 kmph is required.
We will use the Z-score formula to solve this problem.
Z-score formula:
Z = (X - μ) / σWhereX = 80 (the speed we are interested in)
μ = 85 (mean of the normal distribution)
σ = 5 (standard deviation of the normal distribution)
Z = (80 - 85) / 5 = -1
The Z-score for the speed of 80 kmph is -1.
Using the standard normal distribution table,
we find that the probability that the Z-score is less than -1 is 0.1587 (rounded to four decimal places).
For similar question on probability.
https://brainly.com/question/7965468
#SPJ11
Olivia bought snacks for her team's practice. She bought a bag of apples for $3.19 and a 24-pack of juice bottles. The total cost before tax was $55.51. Which tape diagram could be used to represent the context if
�
x represents how much each bottle of juice costs?
Answers
The expression 24x = 55.51 - 3.19 the given condition and the number of bottle is 2.18 or 2 option D is correct
Given that,
She bought a bag of apple for $3.19 and a 24-pack of juice bottles. The total cost before tax was $55.51.
To determine the diagram could be used to represent the context if x represents how much each bottle of juice costs.
What is arithmetic?
In mathematics, it deals with numbers of operations according to the statements. There are four major arithmetic operators, addition, subtraction, multiplication, and division,
Here,
Let the cost of each juice bottle be x.
Now,
According to the question,
the expression will be
24x = 55.51 - 3.19
x= 2.18
Learn more about arithmetic here:
brainly.com/question/14753192
#SPJ1
Write the equation of the line in standard form.
y = 3x + 10
X
x
X
X
A
B
C
D
3x + y = -10
3x + y = 10
3x - y = -10
3x - y = 10
Answers
The equation of the line y = 3x + 10 in standard form is 3x - y = -10.
What is the equation of line in standard form?Given the equation of line in the question:
y = 3x + 10
To write the equation of the line in standard form, we need to rearrange the equation so that the x and y terms are on the left side and the constant term is on the right side of the equation.
It is expressed as;
Ax + Bx = C
y = 3x + 10
Subtracting 3x from both sides, we get:
y - 3x = 3x - 3x + 10
y - 3x = 10
Reorder
-3x + y = 10
Multiply each term by -1
-3x(-1) + y(-1) = 10(-1)
3x - y = -10
Therefore, the equation in standard form is 3x - y = -10.
Learn more about equation of line here:https://brainly.com/question/28696396
#SPJ1
The distance from the center of the circle to any point on the edge of the circle. Responses a) Radius b) Circumference c) Diameter d) Tangent Line
Answers
Answer:
B. Radius
The line from the center of a circle to the edge is called a radius. The radius can be used in pi and a lot of other things
Do the Cauchy-Schwarz Inequality and the triangle inequality hold for the given vectors and inner product?u = (5, 3), v = (14, 4), (u, v) = u · v(a) the Cauchy-Schwarz Inequality Yes / No(b) the triangle inequality Yes / No
Answers
a) Yes, the Cauchy-Schwarz Inequality holds for the given vectors and inner product. Since 74 ≤ 76.84, the Cauchy-Schwarz Inequality holds.
b) Yes, the triangle inequality holds for the given vectors and inner product. Since 20.25 ≤ 23.21, the triangle inequality holds.
How do we know the Cauchy-Schwarz Inequality and the triangle inequality hold given vectors and inner product?Cauchy-Schwarz Inequality:
Using the given inner product, we have:
|u · v| ≤ ||u|| ||v||where ||u|| and ||v|| denote the norms of the vectors u and v, respectively.
We can calculate:
||u|| = sqrt(5^2 + 3^2) = sqrt(34)||v|| = sqrt(14^2 + 4^2) = sqrt(212)Then, applying the Cauchy-Schwarz Inequality, we have:
|u · v| = |(5)(14) + (3)(4)| = 74||u|| ||v|| = sqrt(34) sqrt(212) ≈ 76.84Triangle Inequality:
The triangle inequality states that for any vectors u, v, and w, we have:
||u + v|| ≤ ||u|| + ||v||Using the given vectors, we can calculate:
||u + v|| = ||(5 + 14, 3 + 4)|| = ||(19, 7)|| = sqrt(19^2 + 7^2) ≈ 20.25||u|| + ||v|| = sqrt(5^2 + 3^2) + sqrt(14^2 + 4^2) ≈ 23.21Learn more about Triangle Inequality
brainly.com/question/30298845
#SPJ11
This is for algebra 1 in grade 9. Can anyone help?
Answers
The repair costs $90 for parts, plus $70 per hour for labor describes the situation. The solution has been obtained by using the equation.
What is an equation?
An equation is an assertion that two expressions containing variables and/or numbers are equivalent. The basic driving factors behind the growth of mathematics have been the attempts to rationally answer equations, which are essentially questions.
We are given an equation of the function as C(x) = 90 + 70x.
This depicts that $90 is fixed cost whereas $70 is variable cost and is dependent on the value of x.
So, the statement that describes the given situation is that the he repair costs $90 for parts, plus $70 per hour for labor.
Hence, the fourth option is the correct option.
Learn more about equation from the given link
brainly.com/question/25976025
#SPJ1
joe scored in the 20th percentile on a standardized test. he brags to his friends that he scored better than 80% of the people who took the test. joe is .
Answers
Joe is incorrect in claiming that he scored better than 80% of the people who took the test.
The 20th percentile means that Joe's score was equal to or greater than 20% of the other test takers, but lower than 80%. The percentile is not a percentage, so the statement Joe made is false.
It is important to understand that percentile rankings are not the same as percentages. Percentile rankings measure how one’s score compares to others who have taken the same test. For example, if Joe scored in the 20th percentile, it means that 20% of the other test takers had the same or lower scores. On the other hand, percentages measure a proportion of the whole. In Joe's case, 80% would mean that 80 out of 100 test takers had a higher score than Joe.
To be more accurate, Joe could have said that he scored better than 20% of the people who took the test. Percentile rankings are often used to measure an individual's performance in comparison to a larger group of peers. Although Joe might have performed well, it is important to understand the difference between percentile and percentage.
See more about percentile at: https://brainly.com/question/27903388
#SPJ11